L/R Time Constant

The current curve is exponential as shown in Figure 13.3.

  • The final current is V/R.

  • The voltage across the inductor is expected to fall off exponentially with time. The current rises to 63% in one time constant.

  • The ratio = L/R has units of time and is a time constant.

  • As value of the resistance decreases, the value of ? will increase.

  • For example consider an ideal inductor with no internal resistance, and a zero external resistance ®, will be very large.

  • This means that once a current is established, it continues to flow and never diminishes.

  • Practically this situation exists when the conductor in the inductor is superconductive.

  • This continuous current flow represents stored magnetic field energy

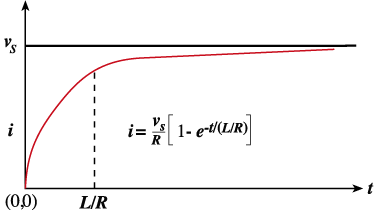
Figure 13.3 Exponential charging curve of an inductor.


Applications of inductors

Applications using inductors are less common than those using capacitors, but inductors are very common in high frequency circuits. One of the major applications of inductors come in from mutually coupled coils where the magnetic field established in one coil cuts through the other coil and hence induces a voltage in the other coil. This is called mutual inductance. Such coils are widely used in transformers.

               

Content and Pedagogy© 2004, University of Ottawa, Faculty of Engineering and Faculty of Education
Design and Production © 2004, University of Ottawa, Centre for e-Learning